In the simplest ocean models the ocean surface is modeled as a pressure release
surface and the ocean bottom is assumed perfectly rigid. This leads to
Dirichlet and Neumann boundary conditions
respectively and the modal problem is
a conventional Sturm-Liouville eigenvalue problem. Considering the bottom
boundary we note that there really is no well-defined bottom depth--- below
sediment lies basalt and one may continue from mantle to core, ... The
truncation of the interval is justified when including additional depth no
longer results in a significant change in the result. This somewhat nebulous
transition occurs when the ocean subbottom is thick enough that material
absorption eliminates significant energy return from deeper depths by
refraction or reflection.
Mitigating against a conservative policy in carrying depth varying properties to great depths is the increased cost of solving the modal equation on a large domain. Thus it is desirable to truncate the problem at the shallowest possible depth. The rigid bottom model makes sense at a sediment/basalt interface where there is a strong impedance contrast. Basalts, however, are typically characterized by a strong elastic wave speed gradient which refracts ray paths back into the ocean. At mid- to high-frequencies, say above 50 Hz, this refracted energy will be severely attenuated and may be safely ignored. A more realistic bottom boundary condition is obtained with an acoustic half-space.
The boundary conditions corresponding to these various cases are
provided below. The results are presented in three forms: 1) as a Robin
condition on the pressure, 2) as boundary conditions on the
stress-displacement vector 
and 3) as boundary conditions on
the solvability vector 
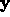. The first form is used for problems
where all internal media are acoustic; the second form is used for
problems where some internal media are elastic; the third form is used
when elastic displacements are required ( KRAKEL
).