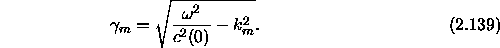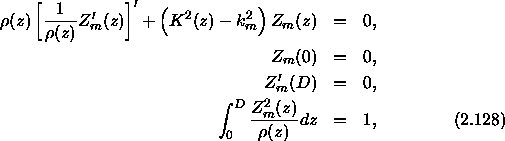
Loss may be caused by scatter at boundaries or interfaces or by material absorption. In the former case the loss is manifest as a complex sound speed while in the latter case the interfacial condition is modified. Both of these mechanisms can be handled by straightforward modifications of the numerical algorithm; however, the eigenvalues become complex, requiring the use of complex arithmetic. More importantly, the root-finder must be modified to perform not just a line search on the real axis but a 2-D search in the complex k-plane. While robust and efficient root-finders can be constructed for the real problem, the complex root-finders are failure-prone.
An attractive alternative to complex eigenvalue searches is to compute the real eigenvalues and then obtain an approximation to the imaginary parts using perturbation theory. To illustrate the technique we consider the modal problem with simple pressure-release and rigid-bottom boundary conditions. That is,
where,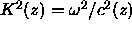 
.
We next write
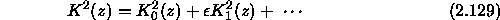
where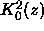 
corresponds to the unperturbed sound speed profile
which for lossy problems is simply the real part of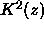 
. In the
following the subscript indicating mode number will be suppressed.
The next step is to seek a solution of the form:
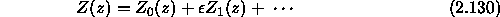
and
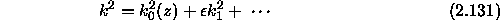
Substituting into Eq. (
) and collecting terms of like order we
obtain:
This is the lossless eigenvalue problem and can be solved on the real axis. The next higher-order equation is,
From the Fredholm Alternative Theorem, the inhomogeneous term on the
right-hand side must be orthogonal to all solutions of the
homogeneous adjoint problem in order for a solution to
exist[41]. (In terms of a vibrating string, this means
that a steady-state solution does not exist when the string is
forced at a resonant frequency.) The solutions of the adjoint
problem are simply the modes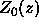 
.
Therefore, we must have
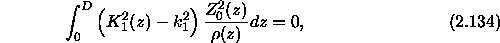
which implies,
This is the first correction due to an arbitrary perturbation
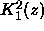. To apply this formula to material absorption we take the
complex sound speed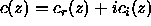 
and form 
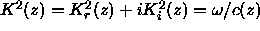. The real part, 
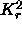, is
used to generate our zeroth-order unperturbed problem which is easily
solved to provide eigenvalues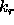 
and eigenfunctions 
. We
next denote the perturbation term by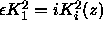 
and the corresponding perturbation to the eigenvalue by 
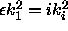. In this notation, Eq. () reads
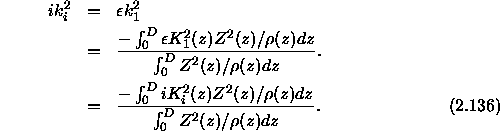
or,
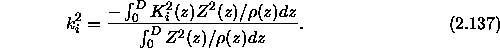
In practice, this perturbational approximation is usually adequate. It gives poor numerical accuracy when the imaginary part is very large, that is, when the mode decays very rapidly in range. In such cases the accuracy is normally not critical since the pressure field is then usually dominated by other modes which are less severely attenuated.
A similar perturbational approach is also useful for treating loss due to surface or bottom roughness. Kuperman and Ingenito[49] derive the result:
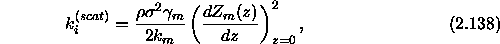
for the perturbation in the eigenvalues due to surface scatter loss. Here,
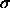denotes the RMS roughness of the sea surface, and
